Regras do fórum
A classificação destes desafios em fáceis, médios e difíceis, é apenas ilustrativa.
Eventualmente, o que pode ser difícil para a maioria, pode ser fácil para você e vice-versa.
 por Balanar » Sáb Ago 07, 2010 17:58
por Balanar » Sáb Ago 07, 2010 17:58
IME-96
Seja f uma função real tal que
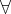
a
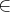

![f(x+a)=\frac{1}{2}+\sqrt[]{f(x)-{\left[ f(x) \right]}^{2}}} f(x+a)=\frac{1}{2}+\sqrt[]{f(x)-{\left[ f(x) \right]}^{2}}}](/latexrender/pictures/ea892c68adbdd818a1fec22c9e3b64cf.png)
, f é periódica?
Justifique:
Resposta:
f é periódica de período 2a.
-
Balanar
- Usuário Parceiro

-
- Mensagens: 72
- Registrado em: Qua Dez 03, 2008 07:18
- Formação Escolar: ENSINO MÉDIO
- Andamento: cursando
 por Douglasm » Sáb Ago 28, 2010 17:02
por Douglasm » Sáb Ago 28, 2010 17:02
Para que uma função seja periódica, deve ser válida a seguinte igualdade:
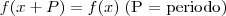
Temos, portanto, que tentar expressar a função desejada do modo acima. Começaremos organizando-a de outro modo:
![f(x+a) = \frac{1}{2} + \sqrt{f(x) - [f(x)]^2} \;\therefore f(x+a) = \frac{1}{2} + \sqrt{f(x) - [f(x)]^2} \;\therefore](/latexrender/pictures/8369cc957a4a379a926adfa8ad9a81fd.png)
![\left[f(x+a) - \frac{1}{2}\right]^2 = f(x) - [f(x)]^2 \;\therefore \left[f(x+a) - \frac{1}{2}\right]^2 = f(x) - [f(x)]^2 \;\therefore](/latexrender/pictures/67934cfc9b0bbec125b94f767a014717.png)
![\left[f(x+a) - \frac{1}{2}\right]^2 = \frac{1}{4} - \frac{1}{4} + f(x) - [f(x)]^2 \;\therefore \left[f(x+a) - \frac{1}{2}\right]^2 = \frac{1}{4} - \frac{1}{4} + f(x) - [f(x)]^2 \;\therefore](/latexrender/pictures/14148f48f94826039e9a56c7ce51745d.png)
![\left[f(x+a) - \frac{1}{2}\right]^2 = \frac{1}{4} - \left[f(x) - \frac{1}{2}\right]^2 \;\fbox{1} \left[f(x+a) - \frac{1}{2}\right]^2 = \frac{1}{4} - \left[f(x) - \frac{1}{2}\right]^2 \;\fbox{1}](/latexrender/pictures/69cdded71ee4f383679fef1089e80d0e.png)
Para facilitar, vamos fazer a seguinte substituição:
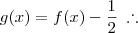
![\left[g(x+a)\right]^2 = \frac{1}{4} - \left[g(x)\right]^2 \left[g(x+a)\right]^2 = \frac{1}{4} - \left[g(x)\right]^2](/latexrender/pictures/5bc54eb052657fe457d327269dd512f7.png)
Se agora considerarmos a função para
x = x + a, teremos:
![\left[g(x+2a)\right]^2 = \frac{1}{4} - \left[g(x + a)\right]^2 \;\therefore \left[g(x+2a)\right]^2 = \frac{1}{4} - \left[g(x + a)\right]^2 \;\therefore](/latexrender/pictures/d7a0bf58ea4ccbea0cc42e1cc289aa0d.png)
![\left[g(x+2a)\right]^2 = \frac{1}{4} - \frac{1}{4} + \left[g(x)\right]^2 \;\therefore \left[g(x+2a)\right]^2 = \frac{1}{4} - \frac{1}{4} + \left[g(x)\right]^2 \;\therefore](/latexrender/pictures/258ee2672309e983bba4e4d8bc92be20.png)
![\left[g(x+2a)\right]^2 = \left[g(x)\right]^2 \;\therefore \left[g(x+2a)\right]^2 = \left[g(x)\right]^2 \;\therefore](/latexrender/pictures/3d8cabb06d1504c04b8d98621185d0ed.png)

Para justificar o último passo, note (através de
1) que:

Consequentemente:

Isso é o bastante para justificar que
g(x+2a) = g(x). Como
g(x) = f(x) - 1/2 , analisando graficamente, notamos que o termo
-1/2 só desloca o gráfico de
f(x) sem alterar sua forma, tampouco sua periodicidade. Demonstramos então que
f(x) é uma função periódica cujo período é
2a.
-

Douglasm
- Colaborador Voluntário

-
- Mensagens: 270
- Registrado em: Seg Fev 15, 2010 10:02
- Formação Escolar: ENSINO MÉDIO
- Andamento: formado
 por Balanar » Sáb Ago 28, 2010 17:50
por Balanar » Sáb Ago 28, 2010 17:50
Brilhante resposta, parabéns.
-
Balanar
- Usuário Parceiro

-
- Mensagens: 72
- Registrado em: Qua Dez 03, 2008 07:18
- Formação Escolar: ENSINO MÉDIO
- Andamento: cursando
 por Douglasm » Sáb Ago 28, 2010 17:59
por Douglasm » Sáb Ago 28, 2010 17:59
Que nada, eu já conhecia esse tipo de questão. =)
-

Douglasm
- Colaborador Voluntário

-
- Mensagens: 270
- Registrado em: Seg Fev 15, 2010 10:02
- Formação Escolar: ENSINO MÉDIO
- Andamento: formado
 por kamillanjb » Ter Mar 15, 2011 22:57
por kamillanjb » Ter Mar 15, 2011 22:57
MAs tá muito bem explicado. Sério mesmo. De grande ajuda.
-
kamillanjb
- Usuário Ativo

-
- Mensagens: 15
- Registrado em: Qua Fev 16, 2011 10:18
- Formação Escolar: ENSINO MÉDIO
- Andamento: formado
Voltar para Desafios Difíceis
Se chegou até aqui, provavelmente tenha interesse pelos tópicos relacionados abaixo.
Aproveite a leitura. Bons estudos!
-
- P.G. Dízima Periódica
por Rafael16 » Qua Jul 18, 2012 19:48
- 3 Respostas
- 8038 Exibições
- Última mensagem por Russman

Qua Jul 18, 2012 21:20
Progressões
-
- Dizima periodica composta
por creberson » Sex Mai 24, 2019 11:03
- 1 Respostas
- 9511 Exibições
- Última mensagem por DanielFerreira

Qui Set 12, 2019 23:19
Conjuntos
-
- [Sequência convergente e periódica ] Prove ...
por e8group » Seg Jan 20, 2014 23:45
- 0 Respostas
- 8388 Exibições
- Última mensagem por e8group

Seg Jan 20, 2014 23:45
Sequências
-
- Função real definida pela soma de uma função par c/uma ímpar
por Taah » Sáb Mar 27, 2010 15:33
- 3 Respostas
- 5195 Exibições
- Última mensagem por Taah

Dom Mar 28, 2010 13:21
Funções
-
- [plano tangente a função de duas variaveis dada por função]
por isaac naruto » Qui Dez 31, 2015 16:35
- 0 Respostas
- 4328 Exibições
- Última mensagem por isaac naruto

Qui Dez 31, 2015 16:35
Cálculo: Limites, Derivadas e Integrais
Usuários navegando neste fórum: Nenhum usuário registrado e 2 visitantes
Assunto:
Princípio da Indução Finita
Autor:
Fontelles - Dom Jan 17, 2010 14:42
Não sei onde este tópico se encaixaria. Então me desculpem.
Eu não entendi essa passagem, alguém pode me explicar?

O livro explica da seguinte forma.
1°) P(1) é verdadeira, pois

2°) Admitamos que

, seja verdadeira:
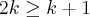
(hipótese da indução)
e provemos que

Temos: (Nessa parte)

Assunto:
Princípio da Indução Finita
Autor:
MarceloFantini - Seg Jan 18, 2010 01:55
Boa noite Fontelles.
Não sei se você está familiarizado com o
Princípio da Indução Finita, portanto vou tentar explicar aqui.
Ele dá uma equação, no caso:

E pergunta: ela vale para todo n? Como proceder: no primeiro passo, vemos se existe pelo menos um caso na qual ela é verdadeira:

Portanto, existe pelo menos um caso para o qual ela é verdadeira. Agora, supomos que
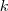
seja verdadeiro, e pretendemos provar que também é verdadeiro para

.

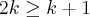


Daí pra frente, ele usou o primeiro membro para chegar em uma conclusão que validava a tese. Lembre-se: nunca saia da tese.
Espero ter ajudado.
Um abraço.
Assunto:
Princípio da Indução Finita
Autor:
Fontelles - Seg Jan 18, 2010 02:28
Mas, Fantini, ainda fiquei em dúvida na passagem que o autor fez (deixei uma msg entre o parêntese).
Obrigado pela ajuda, mesmo assim.
Abraço!
Assunto:
Princípio da Indução Finita
Autor:
Fontelles - Qui Jan 21, 2010 11:32
Galera, ajuda aí!
Por falar nisso, alguém conhece algum bom material sobre o assunto. O livro do Iezzi, Matemática Elementar vol. 1 não está tão bom.
Assunto:
Princípio da Indução Finita
Autor:
MarceloFantini - Qui Jan 21, 2010 12:25
Boa tarde Fontelles!
Ainda não estou certo de qual é a sua dúvida, mas tentarei novamente.
O que temos que provar é isso:

, certo? O autor começou do primeiro membro:

Isso é verdadeiro, certo? Ele apenas aplicou a distributiva. Depois, partiu para uma desigualdade:

Que é outra verdade. Agora, com certeza:

Agora, como
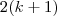
é

a

, e este por sua vez é sempre

que

, logo:

Inclusive, nunca é igual, sempre maior.
Espero (dessa vez) ter ajudado.
Um abraço.
Assunto:
Princípio da Indução Finita
Autor:
Caeros - Dom Out 31, 2010 10:39
Por curiosidade estava estudando indução finita e ao analisar a questão realmente utilizar a desigualdade apresentada foi uma grande sacada para este problema, só queria tirar uma dúvida sobre a sigla (c.q.d), o que significa mesmo?
Assunto:
Princípio da Indução Finita
Autor:
andrefahl - Dom Out 31, 2010 11:37
c.q.d. = como queriamos demonstrar =)
Assunto:
Princípio da Indução Finita
Autor:
Abelardo - Qui Mai 05, 2011 17:33
Fontelles, um bom livro para quem ainda está ''pegando'' o assunto é:'' Manual de Indução Matemática - Luís Lopes''. É baratinho e encontras na net com facilidade. Procura também no site da OBM, vais encontrar com facilidade material sobre PIF... em alguns sites que preparam alunos para colégios militares em geral também tem excelentes materiais.
Assunto:
Princípio da Indução Finita
Autor:
MarceloFantini - Qui Mai 05, 2011 20:05
Abelardo, faz 1 ano que o Fontelles não visita o site, da próxima vez verifique as datas.
Assunto:
Princípio da Indução Finita
Autor:
Vennom - Qui Abr 26, 2012 23:04
MarceloFantini escreveu:Abelardo, faz 1 ano que o Fontelles não visita o site, da próxima vez verifique as datas.
Rpz, faz um ano que o fulano não visita o site, mas ler esse comentário dele enquanto respondia a outro tópico me ajudou. hAUEhUAEhUAEH obrigado, Marcelo. Sua explicação de indução finita me sanou uma dúvida sobre outra coisa.

Powered by phpBB © phpBB Group.
phpBB Mobile / SEO by Artodia.
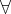 a
a 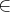

![f(x+a)=\frac{1}{2}+\sqrt[]{f(x)-{\left[ f(x) \right]}^{2}}} f(x+a)=\frac{1}{2}+\sqrt[]{f(x)-{\left[ f(x) \right]}^{2}}}](/latexrender/pictures/ea892c68adbdd818a1fec22c9e3b64cf.png) , f é periódica?
, f é periódica?
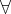 a
a 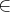

![f(x+a)=\frac{1}{2}+\sqrt[]{f(x)-{\left[ f(x) \right]}^{2}}} f(x+a)=\frac{1}{2}+\sqrt[]{f(x)-{\left[ f(x) \right]}^{2}}}](/latexrender/pictures/ea892c68adbdd818a1fec22c9e3b64cf.png) , f é periódica?
, f é periódica?
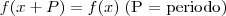
![f(x+a) = \frac{1}{2} + \sqrt{f(x) - [f(x)]^2} \;\therefore f(x+a) = \frac{1}{2} + \sqrt{f(x) - [f(x)]^2} \;\therefore](/latexrender/pictures/8369cc957a4a379a926adfa8ad9a81fd.png)
![\left[f(x+a) - \frac{1}{2}\right]^2 = f(x) - [f(x)]^2 \;\therefore \left[f(x+a) - \frac{1}{2}\right]^2 = f(x) - [f(x)]^2 \;\therefore](/latexrender/pictures/67934cfc9b0bbec125b94f767a014717.png)
![\left[f(x+a) - \frac{1}{2}\right]^2 = \frac{1}{4} - \frac{1}{4} + f(x) - [f(x)]^2 \;\therefore \left[f(x+a) - \frac{1}{2}\right]^2 = \frac{1}{4} - \frac{1}{4} + f(x) - [f(x)]^2 \;\therefore](/latexrender/pictures/14148f48f94826039e9a56c7ce51745d.png)
![\left[f(x+a) - \frac{1}{2}\right]^2 = \frac{1}{4} - \left[f(x) - \frac{1}{2}\right]^2 \;\fbox{1} \left[f(x+a) - \frac{1}{2}\right]^2 = \frac{1}{4} - \left[f(x) - \frac{1}{2}\right]^2 \;\fbox{1}](/latexrender/pictures/69cdded71ee4f383679fef1089e80d0e.png)
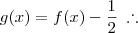
![\left[g(x+a)\right]^2 = \frac{1}{4} - \left[g(x)\right]^2 \left[g(x+a)\right]^2 = \frac{1}{4} - \left[g(x)\right]^2](/latexrender/pictures/5bc54eb052657fe457d327269dd512f7.png)
![\left[g(x+2a)\right]^2 = \frac{1}{4} - \left[g(x + a)\right]^2 \;\therefore \left[g(x+2a)\right]^2 = \frac{1}{4} - \left[g(x + a)\right]^2 \;\therefore](/latexrender/pictures/d7a0bf58ea4ccbea0cc42e1cc289aa0d.png)
![\left[g(x+2a)\right]^2 = \frac{1}{4} - \frac{1}{4} + \left[g(x)\right]^2 \;\therefore \left[g(x+2a)\right]^2 = \frac{1}{4} - \frac{1}{4} + \left[g(x)\right]^2 \;\therefore](/latexrender/pictures/258ee2672309e983bba4e4d8bc92be20.png)
![\left[g(x+2a)\right]^2 = \left[g(x)\right]^2 \;\therefore \left[g(x+2a)\right]^2 = \left[g(x)\right]^2 \;\therefore](/latexrender/pictures/3d8cabb06d1504c04b8d98621185d0ed.png)









 , seja verdadeira:
, seja verdadeira: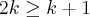 (hipótese da indução)
(hipótese da indução)



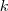 seja verdadeiro, e pretendemos provar que também é verdadeiro para
seja verdadeiro, e pretendemos provar que também é verdadeiro para  .
.
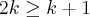


 , certo? O autor começou do primeiro membro:
, certo? O autor começou do primeiro membro:


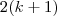 é
é  a
a  , e este por sua vez é sempre
, e este por sua vez é sempre  que
que  , logo:
, logo:
