 por andersontricordiano » Seg Jan 16, 2012 19:46
por andersontricordiano » Seg Jan 16, 2012 19:46
Para que valores reais de m a equação matricial A * X = B em que ,

,

e
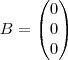
admite uma única solução

-
andersontricordiano
- Colaborador Voluntário

-
- Mensagens: 192
- Registrado em: Sex Mar 04, 2011 23:02
- Formação Escolar: ENSINO MÉDIO
- Andamento: formado
 por Arkanus Darondra » Seg Jan 16, 2012 20:37
por Arkanus Darondra » Seg Jan 16, 2012 20:37
Olá andersontricordiano.
Você tem a resposta do gabarito? Encontrei

mas não tenho certeza.
-
Arkanus Darondra
- Colaborador Voluntário

-
- Mensagens: 187
- Registrado em: Seg Dez 26, 2011 18:19
- Formação Escolar: ENSINO MÉDIO
- Andamento: cursando
 por andersontricordiano » Qua Jan 18, 2012 16:01
por andersontricordiano » Qua Jan 18, 2012 16:01
sim a resposta é X diferente de 4
-
andersontricordiano
- Colaborador Voluntário

-
- Mensagens: 192
- Registrado em: Sex Mar 04, 2011 23:02
- Formação Escolar: ENSINO MÉDIO
- Andamento: formado
 por Arkanus Darondra » Qua Jan 18, 2012 23:52
por Arkanus Darondra » Qua Jan 18, 2012 23:52
andersontricordiano escreveu:Para que valores reais de m a equação matricial A * X = B em que ,

,

e
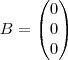
admite uma única solução

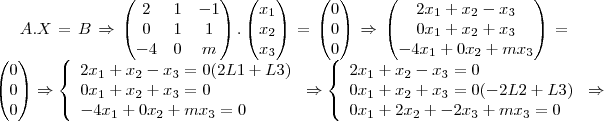

Para o sistema possuir uma única solução, ele deve ser um SPD, portanto, a última linha não pode ser nula.

e

Como ele só pode os valor de

-
Arkanus Darondra
- Colaborador Voluntário

-
- Mensagens: 187
- Registrado em: Seg Dez 26, 2011 18:19
- Formação Escolar: ENSINO MÉDIO
- Andamento: cursando
Voltar para Matrizes e Determinantes
Se chegou até aqui, provavelmente tenha interesse pelos tópicos relacionados abaixo.
Aproveite a leitura. Bons estudos!
-
- [Calcule o valor de Seno de 18º] Expressar o valor numérico
por VictorFPS » Sáb Fev 14, 2015 20:01
- 1 Respostas
- 3705 Exibições
- Última mensagem por adauto martins

Qui Fev 19, 2015 15:41
Trigonometria
-
- Equação matricial
por SAAMS » Dom Nov 28, 2010 17:18
- 0 Respostas
- 3603 Exibições
- Última mensagem por SAAMS

Dom Nov 28, 2010 17:18
Matrizes e Determinantes
-
- Equação matricial ????
por thyerry01 » Qui Set 26, 2013 02:06
- 2 Respostas
- 17420 Exibições
- Última mensagem por thyerry01

Sex Set 27, 2013 01:48
Matrizes e Determinantes
-
- Calcule o valor de x
por thadeu » Ter Jun 29, 2010 20:26
- 2 Respostas
- 7436 Exibições
- Última mensagem por thadeu

Ter Jun 29, 2010 21:29
Piadas
-
- Calcule o valor de x
por andersontricordiano » Seg Fev 24, 2014 16:09
- 1 Respostas
- 1632 Exibições
- Última mensagem por Man Utd

Seg Fev 24, 2014 17:04
Números Complexos
Usuários navegando neste fórum: Nenhum usuário registrado e 2 visitantes
Assunto:
[Função] do primeiro grau e quadratica
Autor:
Thassya - Sáb Out 01, 2011 16:20
1) Para que os pontos (1,3) e (-3,1) pertençam ao grafico da função f(X)=ax + b ,o valor de b-a deve ser ?
2)Qual o maior valor assumido pela função f : [-7 ,10] em R definida por f(x) = x ao quadrado - 5x + 9?
3) A função f, do primeiro grau, é definida pos f(x)= 3x + k para que o gráfico de f corte o eixo das ordenadas no ponto de ordenada 5 é?
Assunto:
[Função] do primeiro grau e quadratica
Autor:
Neperiano - Sáb Out 01, 2011 19:46
Ola
Qual as suas dúvidas?
O que você não está conseguindo fazer?
Nos mostre para podermos ajudar
Atenciosamente
Assunto:
[Função] do primeiro grau e quadratica
Autor:
joaofonseca - Sáb Out 01, 2011 20:15
1)Dados dois pontos A=(1,3) e B=(-3,1) de uma reta, é possivel definir a sua equação.


Em

substitui-se
m, substitui-se
y e
x por um dos pares ordenados, e resolve-se em ordem a
b.
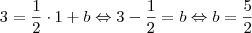
2)Na equação

não existem zeros.Senão vejamos
Completando o quadrado,

As coordenadas do vertice da parabola são

O eixo de simetria é a reta

.Como se pode observar o vertice está acima do eixo Ox, estando parabola virada para cima, o vertice é um mínimo absoluto.Então basta calcular a função para os valores dos extremos do intervalo.
f(-7)=93
f(10)=59
Powered by phpBB © phpBB Group.
phpBB Mobile / SEO by Artodia.
 ,
,  e
e 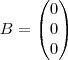 admite uma única solução
admite uma única solução 

 ,
,  e
e 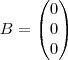 admite uma única solução
admite uma única solução 

 mas não tenho certeza.
mas não tenho certeza.

,
e
admite uma única solução
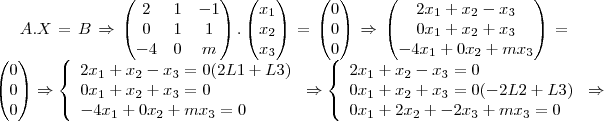

 e
e 




 substitui-se m, substitui-se y e x por um dos pares ordenados, e resolve-se em ordem a b.
substitui-se m, substitui-se y e x por um dos pares ordenados, e resolve-se em ordem a b.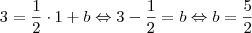
 não existem zeros.Senão vejamos
não existem zeros.Senão vejamos

 .Como se pode observar o vertice está acima do eixo Ox, estando parabola virada para cima, o vertice é um mínimo absoluto.Então basta calcular a função para os valores dos extremos do intervalo.
.Como se pode observar o vertice está acima do eixo Ox, estando parabola virada para cima, o vertice é um mínimo absoluto.Então basta calcular a função para os valores dos extremos do intervalo.