 por JoaoGabriel » Sáb Set 04, 2010 12:01
por JoaoGabriel » Sáb Set 04, 2010 12:01
Resolver em

uma equação exponencial, eu gostaria de saber a maneira correta de igualar bases que são múltiplos entre si.
Ex.1)

Ex.2)
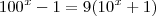
-
JoaoGabriel
- Usuário Ativo

-
- Mensagens: 19
- Registrado em: Qua Ago 18, 2010 16:05
- Formação Escolar: ENSINO MÉDIO
- Área/Curso: Estudando para Engenharia Aeroespacial
- Andamento: cursando
Voltar para Funções
Se chegou até aqui, provavelmente tenha interesse pelos tópicos relacionados abaixo.
Aproveite a leitura. Bons estudos!
-
- Equação exponencial???
por azheng » Sáb Nov 21, 2009 19:47
- 0 Respostas
- 1553 Exibições
- Última mensagem por azheng

Sáb Nov 21, 2009 19:47
Álgebra Elementar
-
- Equação Exponencial
por Adriana Baldussi » Seg Nov 23, 2009 14:41
- 3 Respostas
- 2704 Exibições
- Última mensagem por Molina

Seg Nov 23, 2009 17:07
Álgebra Elementar
-
- Equação Exponencial
por LeonardoSantos » Ter Fev 16, 2010 14:11
- 1 Respostas
- 2711 Exibições
- Última mensagem por Douglasm

Ter Fev 16, 2010 15:46
Funções
-
- Equação exponencial
por cristina » Sex Jun 04, 2010 20:19
- 1 Respostas
- 2165 Exibições
- Última mensagem por Mathmatematica

Sáb Jun 05, 2010 00:27
Sistemas de Equações
-
- Equação exponencial
por nan_henrique » Sáb Jul 10, 2010 13:00
- 1 Respostas
- 2113 Exibições
- Última mensagem por Douglasm

Sáb Jul 10, 2010 13:12
Logaritmos
Usuários navegando neste fórum: Nenhum usuário registrado e 2 visitantes
Assunto:
Exercicios de polinomios
Autor:
shaft - Qua Jun 30, 2010 17:30

Então, o exercicio pede para encontrar

.
Bom, tentei resolver a questão acima desenvolvendo as duas partes em ( )...Logo dps cheguei em um resultado q nao soube o q fazer mais.
Se vcs puderem ajudar !
Assunto:
Exercicios de polinomios
Autor:
Douglasm - Qua Jun 30, 2010 17:53
Bom, se desenvolvermos isso, encontramos:

Para que os polinômios sejam iguais, seus respectivos coeficientes devem ser iguais (ax = bx ; ax² = bx², etc.):


Somando a primeira e a segunda equação:

Finalmente:

Até a próxima.
Powered by phpBB © phpBB Group.
phpBB Mobile / SEO by Artodia.
 uma equação exponencial, eu gostaria de saber a maneira correta de igualar bases que são múltiplos entre si.
uma equação exponencial, eu gostaria de saber a maneira correta de igualar bases que são múltiplos entre si.
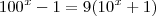

 uma equação exponencial, eu gostaria de saber a maneira correta de igualar bases que são múltiplos entre si.
uma equação exponencial, eu gostaria de saber a maneira correta de igualar bases que são múltiplos entre si.
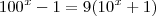

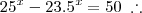

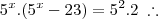

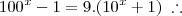

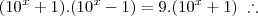
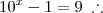



 .
.



