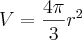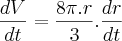por temujin » Qua Mai 29, 2013 17:10
por temujin » Qua Mai 29, 2013 17:10
Boa tarde, pessoal. Essa me pegou...
Dado que

, determine o valor de

para t=0.
O gabarito diz que é 18.
O que eu tentei:


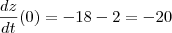
Alguma idéia de onde está o erro??

-
temujin
- Usuário Parceiro

-
- Mensagens: 69
- Registrado em: Qui Mar 14, 2013 15:11
- Formação Escolar: GRADUAÇÃO
- Área/Curso: Economia
- Andamento: formado
 por adauto martins » Seg Out 20, 2014 19:33
por adauto martins » Seg Out 20, 2014 19:33
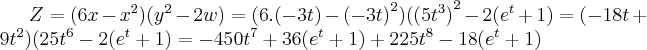
...
dz/dt=

,p/t=0,teremos:
dz/dt=

=18
-
adauto martins
- Colaborador Voluntário

-
- Mensagens: 1171
- Registrado em: Sex Set 05, 2014 19:37
- Formação Escolar: EJA
- Área/Curso: matematica
- Andamento: cursando
 por temujin » Ter Out 21, 2014 17:17
por temujin » Ter Out 21, 2014 17:17
Boa!
Valeu!!


-
temujin
- Usuário Parceiro

-
- Mensagens: 69
- Registrado em: Qui Mar 14, 2013 15:11
- Formação Escolar: GRADUAÇÃO
- Área/Curso: Economia
- Andamento: formado
Voltar para Cálculo: Limites, Derivadas e Integrais
Se chegou até aqui, provavelmente tenha interesse pelos tópicos relacionados abaixo.
Aproveite a leitura. Bons estudos!
-
- Equação Diferencial.
por Higor » Seg Fev 21, 2011 13:12
- 4 Respostas
- 11991 Exibições
- Última mensagem por Higor

Seg Fev 21, 2011 14:46
Cálculo: Limites, Derivadas e Integrais
-
- Equaçao diferencial
por romulo39 » Dom Abr 03, 2011 20:58
- 1 Respostas
- 3809 Exibições
- Última mensagem por LuizAquino

Seg Abr 04, 2011 14:39
Cálculo: Limites, Derivadas e Integrais
-
- Função diferencial
por baianinha » Qua Jun 29, 2011 22:43
- 3 Respostas
- 1821 Exibições
- Última mensagem por LuizAquino

Ter Jul 05, 2011 17:58
Cálculo: Limites, Derivadas e Integrais
-
- Diferencial composto
por Keleber » Sex Out 21, 2011 15:04
- 4 Respostas
- 2410 Exibições
- Última mensagem por LuizAquino

Sáb Out 22, 2011 00:35
Cálculo: Limites, Derivadas e Integrais
-
- [função diferencial]
por Jorge Dias » Sáb Jan 07, 2012 01:08
- 10 Respostas
- 5106 Exibições
- Última mensagem por ant_dii

Ter Jan 10, 2012 00:16
Cálculo: Limites, Derivadas e Integrais
Usuários navegando neste fórum: Nenhum usuário registrado e 2 visitantes
Assunto:
Taxa de variação
Autor:
felipe_ad - Ter Jun 29, 2010 19:44
Como resolvo uma questao desse tipo:
Uma usina de britagem produz pó de pedra, que ao ser depositado no solo, forma uma pilha cônica onde a altura é aproximadamente igual a 4/3 do raio da base.
(a) Determinar a razão de variação do volume em relação ao raio da base.
(b) Se o raio da base varia a uma taxa de 20 cm/s, qual a razão de variação do volume quando o raio mede 2 m?
A letra (a) consegui resolver e cheguei no resultado correto de

Porem, nao consegui chegar a um resultado correto na letra (b). A resposta certa é

Alguem me ajuda? Agradeço desde já.
Assunto:
Taxa de variação
Autor:
Elcioschin - Qua Jun 30, 2010 20:47
V = (1/3)*pi*r²*h ----> h = 4r/3
V = (1/3)*pi*r²*(4r/3) ----> V = (4*pi/9)*r³
Derivando:
dV/dr = (4*pi/9)*(3r²) -----> dV/dr = 4pi*r²/3
Para dr = 20 cm/s = 0,2 m/s e R = 2 m ----> dV/0,2 = (4*pi*2²)/3 ----> dV = (3,2/3)*pi ----> dV ~= 1,066*pi m³/s
Assunto:
Taxa de variação
Autor:
Guill - Ter Fev 21, 2012 21:17
Temos que o volume é dado por:
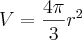
Temos, portanto, o volume em função do raio. Podemos diferenciar implicitamente ambos os lados da equação em função do tempo, para encontrar as derivadas em função do tempo:
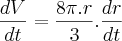
Sabendo que a taxa de variação do raio é 0,2 m/s e que queremos ataxa de variação do volume quando o raio for 2 m:


Powered by phpBB © phpBB Group.
phpBB Mobile / SEO by Artodia.
 , determine o valor de
, determine o valor de  para t=0.
para t=0.

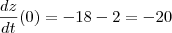


 , determine o valor de
, determine o valor de  para t=0.
para t=0.

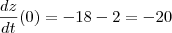


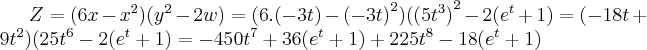 ...
... ,p/t=0,teremos:
,p/t=0,teremos: =18
=18