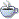por fff » Qua Jan 15, 2014 12:51
por fff » Qua Jan 15, 2014 12:51
Boa tarde. Tenho dúvidas neste exercício na
alínea b. A solução da alínea b é
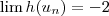
e
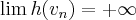

-

fff
- Colaborador Voluntário

-
- Mensagens: 103
- Registrado em: Sáb Dez 21, 2013 11:30
- Formação Escolar: GRADUAÇÃO
- Área/Curso: Engenharia Informática
- Andamento: cursando
 por Guilherme Pimentel » Sex Jan 17, 2014 05:45
por Guilherme Pimentel » Sex Jan 17, 2014 05:45
Definindo:
![h(x)= \left\{
\begin{array}{cc}
\left| \frac{1}{x^2-1}\right|-2 & \textrm{, se }\left| x\right|\neq 1 \\
-1 & \textrm{, se }\left| x\right| =1 \\
\end{array} \right. \\
\textrm{teremos assim:}
\[
\begin{align}
\lim_{x\rightarrow\pm\infty}h(x) &= -2 \\
\lim_{x\rightarrow\pm 1}h(x) &= +\infty \\
h(1)=h(0)&=h(-1)=-1
\end{align}\]\\
\textrm{agora observe que:}
\begin{align}
\lim_{n\rightarrow+\infty}u_n=+\infty &\Rightarrow \lim_{n\rightarrow+\infty}h(u_n)=-2 \\
\lim_{n\rightarrow+\infty}v_{2n}&=1 \\
\lim_{n\rightarrow+\infty}v_{2n+1}&=-1 \\
\textrm{(2) e (3)}&\Rightarrow\lim_{n\rightarrow+\infty}h(v_n)=+\infty
\end{align} h(x)= \left\{
\begin{array}{cc}
\left| \frac{1}{x^2-1}\right|-2 & \textrm{, se }\left| x\right|\neq 1 \\
-1 & \textrm{, se }\left| x\right| =1 \\
\end{array} \right. \\
\textrm{teremos assim:}
\[
\begin{align}
\lim_{x\rightarrow\pm\infty}h(x) &= -2 \\
\lim_{x\rightarrow\pm 1}h(x) &= +\infty \\
h(1)=h(0)&=h(-1)=-1
\end{align}\]\\
\textrm{agora observe que:}
\begin{align}
\lim_{n\rightarrow+\infty}u_n=+\infty &\Rightarrow \lim_{n\rightarrow+\infty}h(u_n)=-2 \\
\lim_{n\rightarrow+\infty}v_{2n}&=1 \\
\lim_{n\rightarrow+\infty}v_{2n+1}&=-1 \\
\textrm{(2) e (3)}&\Rightarrow\lim_{n\rightarrow+\infty}h(v_n)=+\infty
\end{align}](/latexrender/pictures/155a07dd0487c99823c071259c809be7.png)
o gráfico fica:

- Gráfico de h(x)
-
Guilherme Pimentel
- Usuário Ativo

-
- Mensagens: 20
- Registrado em: Dom Jan 12, 2014 19:17
- Formação Escolar: GRADUAÇÃO
- Área/Curso: Matemática/Economia
- Andamento: formado
Voltar para Cálculo: Limites, Derivadas e Integrais
Se chegou até aqui, provavelmente tenha interesse pelos tópicos relacionados abaixo.
Aproveite a leitura. Bons estudos!
-
- [Limites]Preciso de ajuda para calcular alguns limites
por Pessoa Estranha » Ter Jul 16, 2013 17:15
- 2 Respostas
- 4322 Exibições
- Última mensagem por LuizAquino

Qua Jul 17, 2013 09:12
Cálculo: Limites, Derivadas e Integrais
-
- [Limites] Calcular 2 limites notáveis
por fff » Sex Abr 11, 2014 14:26
- 4 Respostas
- 2351 Exibições
- Última mensagem por fff

Sex Abr 11, 2014 19:12
Cálculo: Limites, Derivadas e Integrais
-
- [Limites]Calcular
por fff » Dom Jan 26, 2014 15:08
- 1 Respostas
- 1110 Exibições
- Última mensagem por DanielFerreira

Dom Jul 20, 2014 13:34
Cálculo: Limites, Derivadas e Integrais
-
- [limites]Calcular limite
por fff » Qua Abr 09, 2014 12:29
- 1 Respostas
- 1123 Exibições
- Última mensagem por e8group

Sex Abr 11, 2014 01:14
Cálculo: Limites, Derivadas e Integrais
-
- [Limites] Calcular valor de incógnita
por emanes » Qua Ago 22, 2012 23:33
- 3 Respostas
- 3446 Exibições
- Última mensagem por emanes

Qui Ago 23, 2012 08:46
Cálculo: Limites, Derivadas e Integrais
Usuários navegando neste fórum: Nenhum usuário registrado e 4 visitantes
Assunto:
(FGV) ... função novamente rs
Autor:
my2009 - Qua Dez 08, 2010 21:48
Uma função polinomial f do 1° grau é tal que f(3) = 6 e f(4) = 8.Portanto o valor de f(10) é :
Assunto:
(FGV) ... função novamente rs
Autor: Anonymous - Qui Dez 09, 2010 17:25
Uma função de 1º grau é dada por
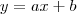
.
Temos que para

,

e para

,

.
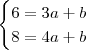
Ache o valor de

e

, monte a função e substitua

por

.
Assunto:
(FGV) ... função novamente rs
Autor:
Pinho - Qui Dez 16, 2010 13:57
my2009 escreveu:Uma função polinomial f do 1° grau é tal que f(3) = 6 e f(4) = 8.Portanto o valor de f(10) é :
f(x)= 2.x
f(3)=2.3=6
f(4)=2.4=8
f(10)=2.10=20
Assunto:
(FGV) ... função novamente rs
Autor:
dagoth - Sex Dez 17, 2010 11:55
isso ai foi uma questao da FGV?
haahua to precisando trocar de faculdade.
Assunto:
(FGV) ... função novamente rs
Autor:
Thiago 86 - Qua Mar 06, 2013 23:11
Saudações!

ví suaquestão e tentei resolver, depois você conta-me se eu acertei.
Uma função de 1º grau é dada por y=3a+b
Resposta :
3a+b=6 x(4)
4a+b=8 x(-3)
12a+4b=24
-12a-3b=-24
b=0
substituindo b na 1°, ttenho que: 3a+b=6
3a+0=6
a=2
substituindo em: y=3a+b
y=30+0
y=30
Powered by phpBB © phpBB Group.
phpBB Mobile / SEO by Artodia.
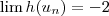 e
e 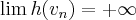


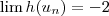 e
e 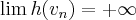


![h(x)= \left\{
\begin{array}{cc}
\left| \frac{1}{x^2-1}\right|-2 & \textrm{, se }\left| x\right|\neq 1 \\
-1 & \textrm{, se }\left| x\right| =1 \\
\end{array} \right. \\
\textrm{teremos assim:}
\[
\begin{align}
\lim_{x\rightarrow\pm\infty}h(x) &= -2 \\
\lim_{x\rightarrow\pm 1}h(x) &= +\infty \\
h(1)=h(0)&=h(-1)=-1
\end{align}\]\\
\textrm{agora observe que:}
\begin{align}
\lim_{n\rightarrow+\infty}u_n=+\infty &\Rightarrow \lim_{n\rightarrow+\infty}h(u_n)=-2 \\
\lim_{n\rightarrow+\infty}v_{2n}&=1 \\
\lim_{n\rightarrow+\infty}v_{2n+1}&=-1 \\
\textrm{(2) e (3)}&\Rightarrow\lim_{n\rightarrow+\infty}h(v_n)=+\infty
\end{align} h(x)= \left\{
\begin{array}{cc}
\left| \frac{1}{x^2-1}\right|-2 & \textrm{, se }\left| x\right|\neq 1 \\
-1 & \textrm{, se }\left| x\right| =1 \\
\end{array} \right. \\
\textrm{teremos assim:}
\[
\begin{align}
\lim_{x\rightarrow\pm\infty}h(x) &= -2 \\
\lim_{x\rightarrow\pm 1}h(x) &= +\infty \\
h(1)=h(0)&=h(-1)=-1
\end{align}\]\\
\textrm{agora observe que:}
\begin{align}
\lim_{n\rightarrow+\infty}u_n=+\infty &\Rightarrow \lim_{n\rightarrow+\infty}h(u_n)=-2 \\
\lim_{n\rightarrow+\infty}v_{2n}&=1 \\
\lim_{n\rightarrow+\infty}v_{2n+1}&=-1 \\
\textrm{(2) e (3)}&\Rightarrow\lim_{n\rightarrow+\infty}h(v_n)=+\infty
\end{align}](/latexrender/pictures/155a07dd0487c99823c071259c809be7.png)



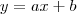 .
. ,
,  e para
e para  ,
,  .
.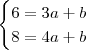
 e
e  , monte a função e substitua
, monte a função e substitua  por
por  .
.