[Geometria Analítica] Vértices do tetraedro

[-civil-]

Boulos - 3a. ed.

18 - 30) O plano contém r : x - y = x + z - 1 = 0 e determina, com os planos coordenados, um tetraedro de volume . Supondo que estamos num sistema ortogonal, obtenha os vértices de tetraedro e uma equação geral de .

Volume do tetraedro =

Consideramos que A está no eixo x, B está no eixo y e C está no eixo z.
A = (x,0,0), B = (0,y,0), C = (0,0,z)
Percebemos que C 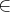r, então C = (0,0,1)

=

=

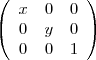= 0
(Na verdade isso é um determinante, só que eu não sei como colocar em Latex)

|xy| =

Como r , então {} é LI

= (x, 0, -1), 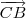= (0, y, -1), = (1, 1, -1)

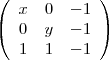= 0


-xy + x + y = 0
Como |xy| =
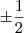
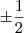+ x + y = 0
x + y =

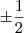
Para x + y = e
Sistema impossível

Para x + y = e

y' = 1, x' =
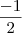
y" = 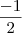, x" = -1

Para x + y = 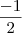e
Sistema impossível

Para x + y = 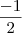e
y' = -1, x' =
y" = , x" = 1

Sem procurar ainda a equação da reta, eu encontrei quatro soluções diferentes. Mas há apenas duas soluções corretas do exercício:

y' = -1, x' =
y" = , x" = 1

Por que as outras duas estão erradas?

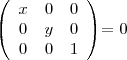
[LuizAquino]

Por que as outras duas estão erradas?

Você está fazendo quatro casos, quando na verdade só há dois.

Da equação |xy| = 1/2, temos dois casos:
(i) xy = 1/2
(ii) xy = -1/2


Quando o caso (i) acontecer, a equação |xy| + x + y = 0 é a mesma que x + y = -1/2.

Por outro lado, quando o caso (ii) acontecer, a equação |xy| + x + y = 0 é a mesma que x + y = 1/2.

(Na verdade isso é um determinante, só que eu não sei como colocar em Latex)

Basta usar o comando:

Código: Selecionar todos

[tex]
\begin{vmatrix}
x & 0 & 0 \\
0 & y & 0 \\
0 & 0 & 1
\end{vmatrix}
[/tex]


O resultado desse comando é: