 por adauto martins » Dom Set 22, 2019 12:47
por adauto martins » Dom Set 22, 2019 12:47
(escola naval-exame de admissao 1938)
verificar que,qualquer que seja

,tem-se:
![arcsen(\sqrt[]{(x/(x+a))}=arctg(\sqrt[]{x/a}) arcsen(\sqrt[]{(x/(x+a))}=arctg(\sqrt[]{x/a})](/latexrender/pictures/e9931fbf4f712c70dbd9b48b96550ba1.png)
-
adauto martins
- Colaborador Voluntário

-
- Mensagens: 1171
- Registrado em: Sex Set 05, 2014 19:37
- Formação Escolar: EJA
- Área/Curso: matematica
- Andamento: cursando
 por adauto martins » Dom Out 06, 2019 16:46
por adauto martins » Dom Out 06, 2019 16:46
soluçao:
façamos
![y=arcsen(\sqrt[]{x/(x+a)}\Rightarrow seny=\sqrt[]{x/(x+a)} y=arcsen(\sqrt[]{x/(x+a)}\Rightarrow seny=\sqrt[]{x/(x+a)}](/latexrender/pictures/96e8919b8cb2dabf27cd256050485b2f.png)
,temos que:
![{seny}^{2}+{cosy}^{2}=1\Rightarrow cosy=\sqrt[]{1-({seny})^{2}}=\sqrt[]{1-x/(x+a)}=\sqrt[]{a/x+a} {seny}^{2}+{cosy}^{2}=1\Rightarrow cosy=\sqrt[]{1-({seny})^{2}}=\sqrt[]{1-x/(x+a)}=\sqrt[]{a/x+a}](/latexrender/pictures/a0a4ddae6af3fac9f0e5fc65db7ab881.png)
,
logo:
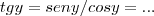
(*) façam os devidos calculos e ...
![y=arctg\sqrt[]{x/a}... y=arctg\sqrt[]{x/a}...](/latexrender/pictures/327acd5a33e697152986d9da905dec3a.png)
-
adauto martins
- Colaborador Voluntário

-
- Mensagens: 1171
- Registrado em: Sex Set 05, 2014 19:37
- Formação Escolar: EJA
- Área/Curso: matematica
- Andamento: cursando
Voltar para Trigonometria
Se chegou até aqui, provavelmente tenha interesse pelos tópicos relacionados abaixo.
Aproveite a leitura. Bons estudos!
-
- exerc.proposto
por adauto martins » Seg Set 16, 2019 15:41
- 1 Respostas
- 12074 Exibições
- Última mensagem por DanielFerreira

Ter Set 17, 2019 12:53
Equações
-
- exerc.proposto
por adauto martins » Seg Set 16, 2019 15:51
- 1 Respostas
- 12442 Exibições
- Última mensagem por DanielFerreira

Ter Set 17, 2019 12:45
Polinômios
-
- exerc.proposto
por adauto martins » Seg Set 16, 2019 16:02
- 1 Respostas
- 4647 Exibições
- Última mensagem por adauto martins

Qui Set 19, 2019 09:39
Equações
-
- exerc.proposto
por adauto martins » Seg Set 16, 2019 16:09
- 4 Respostas
- 8359 Exibições
- Última mensagem por adauto martins

Sex Out 11, 2019 10:34
Números Complexos
-
- exerc.proposto
por adauto martins » Ter Set 17, 2019 10:30
- 1 Respostas
- 3963 Exibições
- Última mensagem por adauto martins

Seg Set 23, 2019 23:57
Equações
Usuários navegando neste fórum: Nenhum usuário registrado e 2 visitantes
Assunto:
Unesp - 95 Números Complexos
Autor:
Alucard014 - Dom Ago 01, 2010 18:22
(UNESP - 95) Seja L o Afixo de um Número complexo

em um sistema de coordenadas cartesianas xOy. Determine o número complexo b , de módulo igual a 1 , cujo afixo M pertence ao quarto quadrante e é tal que o ângulo LÔM é reto.
Assunto:
Unesp - 95 Números Complexos
Autor:
MarceloFantini - Qui Ago 05, 2010 17:27
Seja

o ângulo entre o eixo horizontal e o afixo

. O triângulo é retângulo com catetos

e

, tal que

. Seja

o ângulo complementar. Então

. Como

, o ângulo que o afixo

formará com a horizontal será

, mas negativo pois tem de ser no quarto quadrante. Se

, então

. Como módulo é um:

.
Logo, o afixo é

.
Powered by phpBB © phpBB Group.
phpBB Mobile / SEO by Artodia.
 ,tem-se:
,tem-se:![arcsen(\sqrt[]{(x/(x+a))}=arctg(\sqrt[]{x/a}) arcsen(\sqrt[]{(x/(x+a))}=arctg(\sqrt[]{x/a})](/latexrender/pictures/e9931fbf4f712c70dbd9b48b96550ba1.png)

 ,tem-se:
,tem-se:![arcsen(\sqrt[]{(x/(x+a))}=arctg(\sqrt[]{x/a}) arcsen(\sqrt[]{(x/(x+a))}=arctg(\sqrt[]{x/a})](/latexrender/pictures/e9931fbf4f712c70dbd9b48b96550ba1.png)

![y=arcsen(\sqrt[]{x/(x+a)}\Rightarrow seny=\sqrt[]{x/(x+a)} y=arcsen(\sqrt[]{x/(x+a)}\Rightarrow seny=\sqrt[]{x/(x+a)}](/latexrender/pictures/96e8919b8cb2dabf27cd256050485b2f.png) ,temos que:
,temos que:![{seny}^{2}+{cosy}^{2}=1\Rightarrow cosy=\sqrt[]{1-({seny})^{2}}=\sqrt[]{1-x/(x+a)}=\sqrt[]{a/x+a} {seny}^{2}+{cosy}^{2}=1\Rightarrow cosy=\sqrt[]{1-({seny})^{2}}=\sqrt[]{1-x/(x+a)}=\sqrt[]{a/x+a}](/latexrender/pictures/a0a4ddae6af3fac9f0e5fc65db7ab881.png) ,
,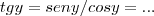
![y=arctg\sqrt[]{x/a}... y=arctg\sqrt[]{x/a}...](/latexrender/pictures/327acd5a33e697152986d9da905dec3a.png)

 em um sistema de coordenadas cartesianas xOy. Determine o número complexo b , de módulo igual a 1 , cujo afixo M pertence ao quarto quadrante e é tal que o ângulo LÔM é reto.
em um sistema de coordenadas cartesianas xOy. Determine o número complexo b , de módulo igual a 1 , cujo afixo M pertence ao quarto quadrante e é tal que o ângulo LÔM é reto. o ângulo entre o eixo horizontal e o afixo
o ângulo entre o eixo horizontal e o afixo  . O triângulo é retângulo com catetos
. O triângulo é retângulo com catetos  e
e  , tal que
, tal que  . Seja
. Seja  o ângulo complementar. Então
o ângulo complementar. Então  . Como
. Como  , o ângulo que o afixo
, o ângulo que o afixo  formará com a horizontal será
formará com a horizontal será  , mas negativo pois tem de ser no quarto quadrante. Se
, mas negativo pois tem de ser no quarto quadrante. Se  , então
, então  . Como módulo é um:
. Como módulo é um:  .
. .
.